Click image for free 18Mb pdf of this "out-of-print" book

This article and others are included in the 18 chapters of "Rethinking the Atom" a 164 page paperback compilation of articles by JMW $19.95 US list at Amazon Click here  for Amazon.com

Click image for free 22Mb pdf of the book

The spdf model was devised for single atoms by physicists and mathematicians. Kowtowing to them, chemists produce hybrid orbitals to explain how atoms could actually form molecules.  Drawing these hybrids and meshing them on paper might look great, but, constrained to measured interatomic physical dimensions and electrostatic interactions, bonding based on the spdf-hybrids (sp, sp2, sp3) is illogical. To have even one electron occupy the “bond” region between the nuclei of diatomic molecules, at the expense of reduced coverage elsewhere, does not make sense for stable molecules. To have two repelling electrons in the area is nonsensical. To a third object, the perceived influence of two electrons may be additive, but the influence perceived by those two electrons of each other will hardly be congenial or neutral, as implied in the “duality” concept! While some question whether electrons are particulate, I have chosen to accept the fact that electrons have mass and, if the mass is a string, for example, it must at least be a ball of twine when fired at something. My qualms have to do with the spdf model and the history of forcing it to “meet” the experimental with mathematics ruling over common application of physical limitations, like e-e repulsion, and chemical properties, like the position of hydrogen in the periodic table. The MCAS model for bonding that is discussed below easily explains why hydrogen has the characteristics of carbon and thus belongs over carbon in the familiar periodic table.[1] This article is about bonding in simple diatomic molecules, however.

The MCAS model will be used to inspect the bonding interactions of simple diatomic molecules. The second period diatomic molecules use just the sp orbitals of the currently accepted spdf model or the M/C orbitals of the MCAS model.[2] The image to the right is an artistic rendition of how the two models place the electron orbitals about the atoms. The spdf model has 7 lobes for an eventual 8 electrons, thus, the requirement to “pair” electrons in the “red” s-orbital – achieved mathematically by spin-reversal. The 8 lobes of the MCAS model easily accommodated 8 electrons with “pairing” by reciprocal motion. For Neon, its 8, second period, electrons are not all uniformly packed around the nucleus with the spdf model. With the MCAS model, they are. Note that for equal orbital extent, the spdf model uses 5.2x the cubic volume of the MCAS model; i.e., the spdf model is not compact as one might expect the electron structure around a nucleus to be. For the purposes of forming diatomic molecules of the second period, the spdf model must be modified to sp, sp², or sp³ hybrids although some MO treatments just make bond-antibond lattices without prehybridizing. No such hybridization is needed with the MCAS model. Since hybridized spdf modeling is so extensively taught in all levels of chemistry text, the reader is assumed to be well acquainted with them.

Atoms of the second period elements cover 8 positions with electrons as they are available. The following figure shows 2 MCAS-model nuclei placed on an axis in close proximity to one another.  

Consider a case wherein 8 electrons fill the 8 lobes; such as with a fluoride ion. With all of the orbital lobes filled, the nucleus is symmetrically surrounded with “guarding” electrons. Consequently, it has no unguarded “posts” that need protecting. On the other hand, fluorine atoms have only 7 electrons. So, how can the unguarded “post” (see small “?’s” in the above figure) be protected? Without an available “free electron”, another atom with a similar deficiency participates. The deficiencies merge and a bond is born. There is neither a need to spin-reverse an electron nor to ignore electron-electron repulsion as occurs in the mathematical treatment of the current spdf-hybrid modeling. In the way the orbitals are arranged in this paper, the red ones are “bonding” and the blue ones are “antibonding”. Note that the bonding quartets include the exo-positions.

The figure at the right illustrates the situation with accompanying electrostatic interactions. The electrostatic contours were determined with the nuclei at their experimental distance apart and with the electrons at the orbital extremes. Non-bonding electron charge was distributed uniformly to each lobe. Classical electrostatic attractions and repulsions with were used. The electrostatic images clearly demonstrate the surrounding symmetry of the 8-electron fluoride ion. They also demonstrate the weakness of the bond in the fluorine molecule. There is little negativeness (blue in the figure) protecting the molecule in this area. Consequently, fluorine is a very reactive electron-acceptor. Thus, while “neighboring” atoms may join to lower their individual vulnerabilities, this is inferior to having a full-time electron do the job. The current practice of putting electrons between the nuclei at the expense of de-shielding in other areas may be mathematically attractive, but it is illogical from standard electrostatic interactions. That is why physics had to be different at the atomic level. A bond represents electron-deficiency NOT electron-abundance. Orbital overlap may, however, provide a conduit for transient flow (!) of electrons from the antibonding quartet of one atom to another.

Consider now the bonding in some simple diatom molecules as given by the MCAS model. The figure at the right shows a plot of the experimental bond strength[3] of the diatomic molecules of the second period of the periodic table.  Li-Li has a modest bond strength with a single “bonding” electron on each atom and no antibonding. There is an “antibonding” electron on each Be atom (this gives symmetry to the individual atom). The greater nuclear positive charge attractions for the bonding electrons is countered by greater nuclei repulsion and repelling of the opposite nucleus’ antibonding electron by the nucleus’ bonding electron and the bond is weaker. As nuclear charges increase and more “bonding” electrons surround the nuclei, bond strength increases greatly (note green line). Maximum bond strength is obtained when there are maximum “bonding” electrons and minimum “antibonding” electrons. This occurs with nitrogen. Beyond nitrogen, “antibonding” electrons are added. Increasing interatomic “bonding-antibonding electron repulsion” and increasing repulsion of the nuclei overshadow increasing nucleus-bonding electron attractions. The MCAS model demonstrates the observed results without altering the disposition of the electrons around the nuclei. Contrast this with the ever changing hybridization required for the spdf-model to do the same.

The 1^st ionization potential of a single atom is now addressed. The figure at the right shows the experimental data[4] of the second period elements. Ionization potential is the difference in the energy level of the original state and that of the generated state. The green line indicates ionization from “optimal” bonding-antibonding electron configurations (Be, N, and Ne; all green arrows) to “less optimal” configurations. Red arrows indicate electrons in “non-optimal” configurations. For Li, B, and O, their removal gives an “optimal” configuration. For C and F, removal of an electron from a “non-optimal” configuration just gives another “non-optimal” one. The N-value is slightly lower than expected.

The electron affinity of a single atom and its diatomic molecule is now addressed. The figure at the right shows the experimental data[5] of the second period elements. Consider first the single atom e-affinities (yellow squares and accompanying single dual 4-lobe C-orbitals). Li has an affinity to add an electron to provide symmetry. Adding one to Be destroys its inherent symmetry. Increased nuclear charge and improved symmetry occur with B and C. Adding an electron to N destroys its symmetry which counters its higher nuclear charge. Improved symmetry occurs again with O and F with increased nuclear charge having a dramatic effect. For Ne, the 8-lobes are filled and, consequently, there is no need for an additional electron at this level.

The electron affinity of the diatomic molecules is a bit different (red circle and overlapped C-orbitals in the preceding figure). Li-Li has a slightly lower e-affinity than Li as the addition of an electron to the antibonding lobes would destabilize the Li-Li bond. No data for Be-Be and B-B are given in the reference. The C-C molecule has a much greater e-affinity than atomic C! The N-N molecule would not be expected to have much of a difference in e-affinity than the corresponding single atom which has none. The O-O molecule has a much lower e-affinity than a single O atom. Similarly, the F-F molecule has a lower one than the F atom, but not much lower.

The reason for the deviant electron affinities of the diatomic molecules becomes clear when the electrostatic interactions are considered, especially the enormously greater e-affinity of C-C. The figure at the right shows the calculated positive and negative charge levels around the molecules in the MCAS style with electrons at maximum orbital extension.

First, note that, as the nuclear charge increases (C to Ne), the surround electrons become more tightly bound (compact) and uniform, but always as symmetrical as possible.

Next, note the electrostatics of the diatomic molecules in the bonding area. The images are for 6 bonding electrons between the nuclei; required for N₂ to F₂ as the bonding orbital quartets are filled. For C₂, it is only one of its options which is shown here to emphasize the molecule’s great need for an exo-electron.

The nitrogen diatom is the most uniformly bathed in negativeness. As nuclei-repulsion and interatomic bonding-antibonding repulsions increase, the bond lengthens and bond energy decreases. In the case of F₂, the bond is greatly weakened, even with the nuclei tugging on the opposite’s electrons. At Ne, the need for a bond is replaced by an electron and the atom is more highly bathed in negativeness than is the nitrogen molecule.

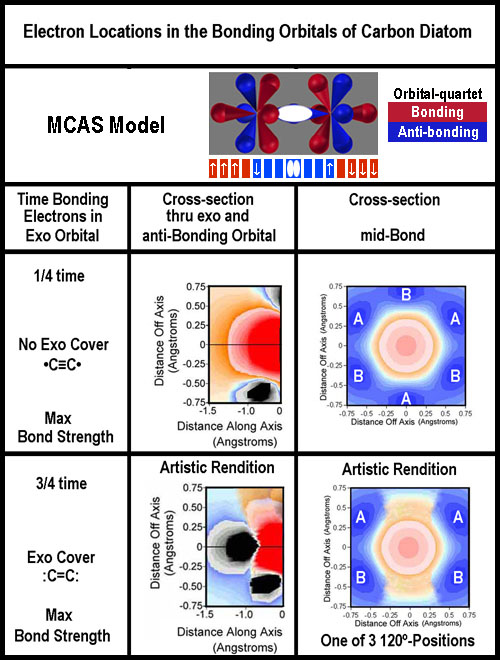Valence bond theory would require 4 bonds between the carbon nuclei to give 8 shared – this was never taught that I remember; but apparently is getting some press[8]. The exo-deficient image above for carbon is like •C≡C• with just 7 electrons for each and the lone electrons anti-bonding, yet paired (by opposite movement).

There are 2 MO versions: sp+2p version (3 bonds between the nuclei; equivalent to the above:  •C≡C•) and s +3p version (2 bonds, thus :C=C:).[6] One of the electrons outside the nuclei pair is in the non-bonding quartet; the other is in the bonding quartet. They are not paired in the same orbital as is usually implied. The MO model lumps the non-bonding together; here that is clearly not the case.

Unlike the cases of N₂, O₂, and F₂, there are only 3 electrons to fill the 4 bonding quartet lobes. They can be placed in 1 of 4 ways with 3 being energetically equivalent. The two different options are shown in the figure to the right. Whether electrons are "paired" or not between the two nuclei will depend on how they move in the bonding quartets.

Does the mid-bond cross-section for the exo-covered option look like 2, side-by-side, A-B π-bonds for the exo coverage? How about 3 A-B π-bonds for the “no exo cover” option? Unlike the spdf/MO model, there is no connection; just proximity.

Now consider the carbon monoxide case. CO has the same number of electrons as does nitrogen: 10. Unlike the case with nitrogen where each atom has the same number, carbon has 4 and oxygen has 6. The figure at the right shows how the electrons are redistributed when the CO molecule is formed. According to the MCAS modeling, an electron from the “antibonding” orbital unit of oxygen is transferred to the “bonding” orbital of carbon. This results in a permanent dipole. With an electron arrangement like that of N₂, CO should have a bond strength attributed to a triple bond; actually it should be stronger (experimentally observed) with the higher charge on oxygen tugging on the carbon bonding electrons. I have included an MO description[7] of what is happening for comparison. The bonding and anti-bonding sigma and pi orbitals/levels are easily formed schematically, if not envisioned physically. Seems like the four 2s electrons form two exo non-bonding orbitals instead of a sigma/anti-sigma pair! Since there are no unpaired electrons, MO requires (Hund’s rule) that two electrons occupy each “bond” orbital. The MCAS model has no such restriction as each orbital has a complementary opposite; thus, there are no “unpaired” electrons, even if they are at opposite ends of the molecule.

Students can learn to follow the teaching and textbook presentations of the spdf/MO model, but there are some serious questions about these models. For example,

• What is the great driving force to make a C-C molecule have much larger e-affinity (~ equal to F atom) than a C-atom in the visual form of the spdf-model?

• Is there really enough space for two permanent electrons in a sigma bond between the two nuclei, even if the electrons could comingle as required in the mathematical treatment? If these electron are located between the nuclei, as claimed in many models, why is there much electrostatic negativity outside the internuclear region as is usually illustrated in the hydrogen molecule case; esp. on the exo-nuclear axis? 

• Does the σ*-bond from the p-orbitals (as indicated in ref [9]) constitute a bond and thus giving rise to a 4th bond? It does cover the molecule's ends. Having the two anti-bond lobes be a paired unit does get around Hund! The MO also gets around the problems that arise with the often used, very simplistic, boiler-plate, MO notations for C₂. These are illustrated in the bottom of the figure as "A traditional MO"! One is left to wonder why C₂ is so unstable when viewing the "4-bond" MO!
  
  
• Where is the overlap that would even provide a π-bond? What make these π-bonds strong? Some have proposed bent-bonds to provide overlap. Loops might  work with reciprocal motion for pairing. Clouds connecting the tops and bottoms of the p-orbitals are also envisioned [9], but what would create e-pairing. Overlap is not implied in simple spdf-modelings when nuclei are placed apart and the p-orbitals are pear-shaped; spherical shaped p-orbital lobes would be better, but that is not what the mathematicians decree to my understanding. What keeps the electrons "floating"? MO modeling just indicates bonds and antibonds are formed without regard to spatial requirements nor much in the way how other than spin-pairing. What is a filled π-bond + a filled anti-π-bond level but two, filled, non-bonding, p-orbitals – one on each atom? What is a filled σ-bond + a filed anti-σ-bond level but two, filled s-orbitals ala helium? What is the repulsion between these filled non-bonding s and p-orbitals? Also, σ/σ* from an s-orbital should definitely be different than σ/σ* from a p-orbital. Hence, the need to have the p-orbitals form a 2-electron σ* MO in two separate lobes at either end of the C-C axis [9] rather than from the two 2-s orbitals. MOs that assume that sp-hybridization occurs before bonding can get around this. Simply indicating that all the electrons of each atom are the same and then placing them in the needed MOs as I have done in several MO diagrams eliminates the problem. Of course, the real problem is determining what MOs are needed rather than where the electrons started. Carbon monoxide clearly demonstrated that electrons in a molecule go where they are needed - just as they do in the atoms of the periodic table [1].
  
  
• C₂ should have two unpaired electrons in the triple bond case (•C≡C•), if Hund’s rule is to be obeyed in the current models; like the top figure on the right? Is the no-sigma bond image logical, as near the bottom of the figure on the right, if there are paired electrons in the exo-bonds, as indicated in the double bond case (:C=C:), which has no unpaired electrons? Or are there two empty p-orbitals with one sigma bond and one π-bond? The filled s-orbital spheres (red) near the bottom are more in line with "traditionally presented MO diagrams". If they look a bit strange, one might consider that the "simple 'serves-all' MO approach" does not serve all! This "red sphere" illustration of anti-bonding negating bonding to yield s-orbitals emphasizes the shortcomings of the simple MO treatment. Shown this way, the 3D imagery clearly indicates that the 2-s orbitals are not involved in the bonding process in accordance with the accompanying MO diagram. The bottom MO might make sense, if, in fact, p-orbitals can form π-bonds; especially 1-electron π-bonds! The reader is sure to find some others. No wonder some teachers might want to forget the Bohr-orb descendants. Of course, some of the learned might want something more tangible than some energy level lines on paper. I did as I tried to understand chemical reaction mechanisms, etc.
  
  
• 3-D images of the bonding and antibonding orbitals should be presented for students to grasp what is being presented in the MO diagrams. The figure above right does not show antibonding sigma and anti-π-bonds. MO for C₂ has an anti-sigma. Where? Seems a lot of extra stuff to explain what is going on, when the MCAS approach does it so uncomplicatedly.

The figure at the right  presents conceptual imagery of the Valence-Bond (VB), Molecular Orbital (MO) and MCAS approaches to the hydrogen molecule. This allows the three to be compared in the simplest molecular case. 

The discussion has being moved to a separate location. Click here.

Serious consideration of spatial placements and e-e repulsions should raise major concerns, indeed, about the spdf-hybrid system and its MO offshoot. The MCAS model, on the other hand, provides a physical representation that does not resort to e-e non-repelling couplets and stresses coverage of the nuclei on all fronts, rears, and sides.

Summary

The MCAS electron orbital model provides a compact orbital arrangement which explains bond strength, 1st ionization potential, and electron affinity behavior of the diatomic molecules of the second period of the periodic table. It does so without hybridizing (reconfiguring) the orbitals as the spdf model is required to do. It does so while obeying classical physics; something the spdf approach has to declare invalid to operate. Hence, the MCAS model demonstrates that classical physics operates down to and includes the electron orbitals nanospace.

[8] http://www.rsc.org/chemistryworld/2013/05/quadruple-bond-carbon-debate-shaik-hoffmann-frenking

[9] http://www.meta-synthesis.com/webbook/39_diatomics/diatomics.html